反应堆压力容器(reactor pressure vessel,RPV)是支撑反应堆内的所有构件、装载反应堆堆芯、容纳一回路冷却剂并维持其压力的承压壳体,是核电站寿命设计中不可拆换的核心部件[1]。RPV钢通常采用具有辐照稳定性和长期可靠性等特点的铁素体合金钢[2]。长期处于高温高压(290℃、16 MPa)服役环境下,RPV钢会出现辐照硬化和辐照脆化等性能退化现象[3,4],严重影响反应堆安全。研究[5,6]发现,RPV钢在中子辐照下产生的高密度、小尺寸(约2 nm)的富Cu析出相具有阻碍位错运动的作用,是造成辐照脆化和辐照硬化的主要原因。然而,实验研究[7,8]发现,RPV钢在0.026和0.051 dpa的中子辐照后,传统透射电镜下没有观察到富Cu析出相,但同样出现了辐照硬化现象。这一现象的产生,极有可能是辐照硬化初期尺寸极小、难以通过传统实验器材观察到的富Cu析出相所造成的。此外,辐照环境下Cu元素析出速率会极大地提升,这与辐照导致点缺陷大量增加密切相关[6]。因此,通过理论模拟研究辐照硬化初期Fe-Cu合金中细小富Cu相的析出行为具有重要意义。
近年来,相场方法在模拟核材料的组织演变方面展现了巨大的潜力[9],国内外学者针对RPV钢的第二相析出行为也进行了一些模拟[10~13]。相场方法基于Ginzburg-Landau理论和连续界面假设,不仅能高效地展现RPV钢富Cu相析出过程的微观组织变化,而且能动态显示析出过程的浓度场变化情况。Molnar等[14]提出了Fe-Cu合金中富Cu相析出的多尺度模拟,并提出了较为泛用的相场模型模拟手段。最近,Zhu等[15]利用相场理论研究了Fe-Cu合金的富Cu析出相从bcc结构向9R结构的转变过程,从晶体学转变的角度考虑了相变过程中的弹性能。赵宝军等[16]利用相场方法研究了Mn含量对Fe-Cu-Mn合金纳米富Cu析出相的影响。Yan等[17]在利用相场研究Fe-Cr合金的两相分离时提出了一种新的包含缺陷浓度的自由能表达形式。然而,相关研究并未系统考虑Fe-Cu合金中富Cu相析出的形核行为,也没有考虑Fe-Cu合金析出行为受辐照条件的影响。前者可能是影响其低剂量辐照下辐照硬化机制的重要因素,后者则是含Cu铁合金工作环境下的必然因素。
基于能量路径(energy path)优化的约束条件弦方法(constrained string method)是研究析出相的临界形核尺寸和最优能量路径的重要方法[18~20]。作为遗传算法的一种分支,弦方法以弦变量作为演化对象。将弦方法用于析出相形核初期的计算,不仅可以体现形核过程中浓度场的动态演变,而且能够再现临界形核过程和最优能量路径的匹配特性。最近,Li等[18,21,22]利用相场模型和约束条件弦方法结合的物理模型对Fe-Cu及Fe-Cu-Mn合金热力学环境下的析出情况进行了模拟,并计算得到了Cu元素的热力学条件下临界形核尺寸及形核能量路径。Zhang等[23,24]较为全面地介绍了相场模型结合约束条件弦方法的计算手段,并在此基础上对二元固体材料相析出过程和临界形核半径进行了模拟计算。以上工作对Fe-Cu合金在辐照条件下的小尺寸富Cu相析出行为的研究提供了可行思路。
本工作基于相场模型和约束弦方法,构建了Fe-Cu合金在辐照条件下的富Cu相析出过程的相场模型,并结合弦方法,使用半隐式Fourier方程[25]对辐照条件下富Cu相形核析出过程的浓度场进行计算,得到了临界形核尺寸和最优能量路径。分别研究了温度、Cu含量、辐照强度3个因素对RPV模型钢Fe-Cu合金中的小尺寸富Cu相的析出行为的影响,计算得到了不同条件下的临界形核半径、形核能量路径、空位形成与分布,并对计算结果进行了定性分析。
1 模型建立
1.1 弦方法简介
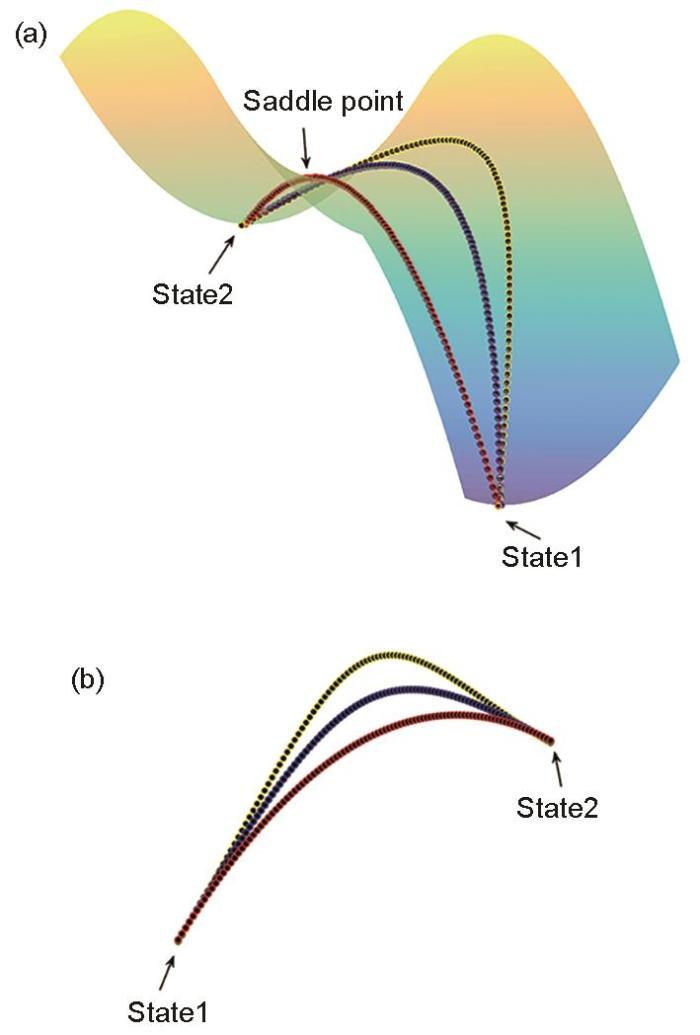
图1所示为第二相析出过程的形核阶段的能量路径变化示意图。图1a中的曲面为在形核过程中形核能的所有可能变化路径形成的曲面示意图,图中的3条曲线为形核过程中形核能随着时间演化的3条能量路径。图1b为图1a中的3条能量路径所对应的能量势垒的示意图。State 1与State 2为形核开始与结束时所对应的稳定状态。图中的黄色能量路径(EP i )为第i次演化的能量路径,用“弦”表示其形核浓度场的变化情况。假设该能量路径及其“弦”上一共有N个状态点,每个状态点用L(i, n)表示(i = 1、2、…、I,其中I为总演化次数;n = 1、2、…、N)。L(i, n)用来描述第i次演化对应的形核过程中第n个状态下的浓度场分布[18,19]。如图1a所示,黄色能量路径所对应“弦”经过演化之后产生的新“弦”为蓝色能量路径所对应的“弦”。蓝色能量路径记为EP i + 1,其“弦”上状态用L(i + 1, n)表示。在演化过程中,黄色“弦”上的每个点均向能量更低的方向移动dL,最后经插值得到蓝色“弦”。由于每个点都是朝着能量更低的方向靠近,因此演化后的“弦”将朝能量更低的方向靠近,“弦”的最高点也逐步降低[19]。经过I轮演化,最终可以获得满足约束条件的最小能量路径,即图中的红色“弦”。红色“弦”的最高点将是所有“弦”各自最高点的最低值,也是整个曲面的“鞍点”。
图1
图1
形核过程鞍点示意图
Fig.1
Schematics of saddle points in the nucleus evolutionary correction process
(a) energy path saddle point diagram(b) energy path height contrast diagram
式中,CCu0为初始设定的Cu元素浓度,CFe为Fe元素浓度,r为空间坐标,ρ为所研究材料的原子密度。
第i轮演化中,非最低能量路径的弦在弦状态L(i, n)的演化表达式为[20]:
式中,E为能量函数,τ为当前能量路径的切向单位向量,dL为能量路径的移动微元。
1.2 相场自由能方程
式中,εCu和εV分别为Cu和空位的本征应变系数;t为时间,t = nΔt,Δt为数值离散格式的时间步长。
| Element | α1 | α2 | α3 | α4 | β | γ | L |
|---|---|---|---|---|---|---|---|
| Cu | 6242.2 | 122.833 | -23.5902 | -0.004761 | -5.919 | 70000 | 38022.8 |
| Fe | 1225.7 | 124.134 | -23.5143 | -0.004397 | -5.892 | 77358.5 | 38022.8 |
表2 Fe-Cu合金的界面能参数与本征应变系数[28,29]
Table 2
| Parameter | Value | Unit |
|---|---|---|
| κCu | 5 × 10-15 | J·m2·mol-1 |
| κV | 1 × 10-15 | J·m2·mol-1 |
| εCu | 122.833 | |
| εV | 124.134 |
1.3 辐照下相场方程的演变
相场理论中浓度场变量的演变方程(Cahn-Hilliard方程)为:
式中,Ci 为所关注对象在i轮演化下的浓度场参数,M为迁移率:
式中,D为扩散系数,气体常量R = 8.314 J/(mol·K)。Cu元素和空位缺陷的扩散系数DCu和DV均可由迁移能[31]得到:
式中,
| T / K | DFe / (m2·s-1) | DCu / (m2·s-1) | MFe / (mol·m·s·kg-1) | MCu / (mol·m·s·kg-1) |
|---|---|---|---|---|
| 550 | 7.2 × 10-32 | 5.0 × 10-29 | 6.7 × 10-17 | 4.6 × 10-14 |
| 650 | 4.2 × 10-27 | 8.0 × 10-25 | 3.9 × 10-12 | 7.4 × 10-10 |
在考虑空位的产生、复合、吸收等作用后,Cu原子和空位浓度的演化方程为[17]:
式中,V为基体中空位的体积。
辐照时将产生大量的点缺陷,从而影响Cu原子的迁移率。研究[35]表明,在较低辐照温度和低空位浓度下,空间迁移率与整体空位浓度成线性相关关系。因此,辐照条件下迁移率MR及扩散系数DR将表示为:
式中,M0为无辐照条件下的迁移率;CVr为辐照额外产生的空位浓度,辐照产生空位浓度与热力学平衡状态空位浓度2者之和为辐照环境的总空位量。根据Odette等[35]的工作,CVr与辐照强度有关:
式中,GV为每原子离位产生的空位数量,ϕdpa为辐照产生的原子离位率。可见,在指定材料中CVr直接由辐照强度确定。
GV取决于原子的临界复合半径rV和Vm。在稳态情况下其值由RED模型[35]求解,解析方程为:
式中,Rt为陷阱的复合半径;τt为空位的捕获时间,其取值为:
式中,kB为Boltzmann常数。
将
式中,B为整合系数:
将
1.4 模型的无量纲化
对相场模型进行参数无量纲化处理,得到标注*号的无量纲化参数。无量纲化过程如下:在无量纲空间,位置坐标r* = r / l0,无量纲时间单位t* = tD / l02,无量纲Cu元素的迁移率M
1.5 模型的数值计算方法
约束条件弦方法通过初始条件规定演化方向,用约束条件限制演化目标。与一般的遗传算法相似,约束条件弦方法也需要构建遗传变量的遗传递推方程。本工作使用快速Fourier变换方法对演化方程进行处理[25],所得到的半隐式Fourier方程形式为:
式中,k为Fourier向量空间坐标。在形核模拟中C(i, t)和C(i + 1, t)分别表示在第i轮演化与第i + 1轮演化中弦变量L上时刻t的状态对应的浓度场变量。
将浓度场变量C(i, t)按照
2 结果与讨论
2.1 最小能量路径与临界形核半径的计算
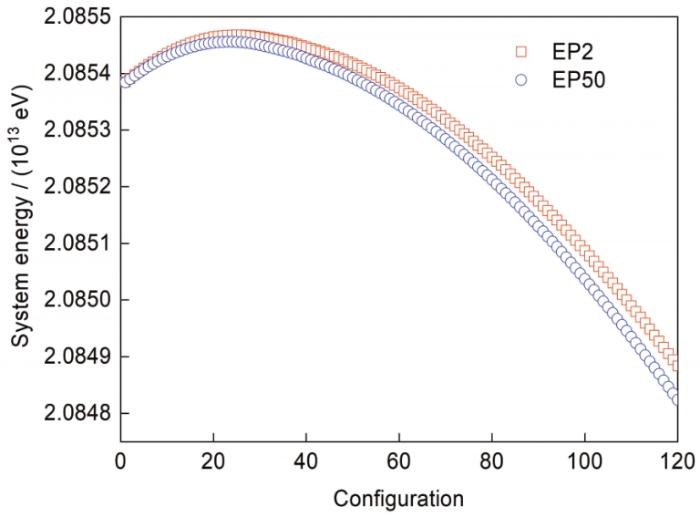
图2为650 K时Fe-0.3%Cu (质量分数)合金中富Cu团簇的非典型形核过程中的能量路径的变化曲线。纵坐标为体系总能量,横坐标为对应状态数[18]。曲线最左端的点对应Cu元素均匀分布的初始态,最高点对应临界富Cu团簇的状态,最右端对应一定演化时间后的状态。本工作中,将形核过程的每根“弦”分割为120个时间步长,单根“弦”上的每个状态数对应一个时间节点。50轮次弦的演化对应50次形核能量路径的演化。可以看出,能量路径是一个先升高后降低的势垒函数,经过多次演化,形核能量路径的最高值不断降低,这符合弦方法的特征[19,20]。本工作中,在弦不断演化的同时,其对应的能量路径不断逼近最小能量路径,最终实现最小能量路径形核过程的计算。到达势垒最高值所对应的时间,即形核半径长大到达临界形核半径所需要的时间。根据
图2
图2
650 K时Fe-0.3%Cu合金在辐照强度0.01 dpa/s下演化2步和50步后的能量路径
Fig.2
Energy paths of Fe-0.3%Cu alloy at 650 K and radiation intensity of 0.01 dpa/s (EP2 for energy path after 2-step-revolution, EP50 for energy path after 50-step-revolution. The abscissa represents the number of nucleus time states, the same in the figures below)
图3
图3
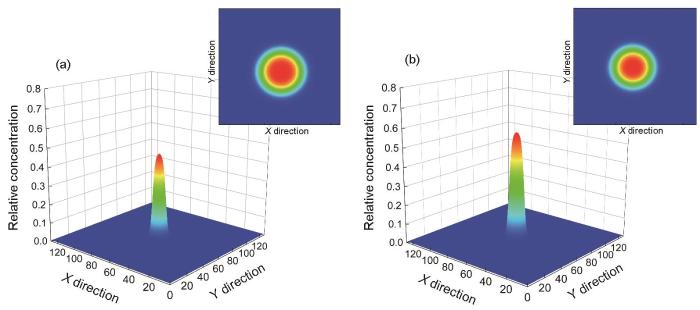
650 K和0.01 dpa/s下Fe-0.3%Cu演化2步和50步后临界团簇的Cu元素浓度分布和团簇形貌
Fig.3
Cu concentration distributions and top views of cluster morphologies of critical nucleus (insets) of Fe-0.3%Cu alloy at 650 K and radiation intensity of 0.01dpa/s
(a) cluster after 2-step-revolution (b) cluster after 50-step-revolution
2.2 温度对形核过程的影响
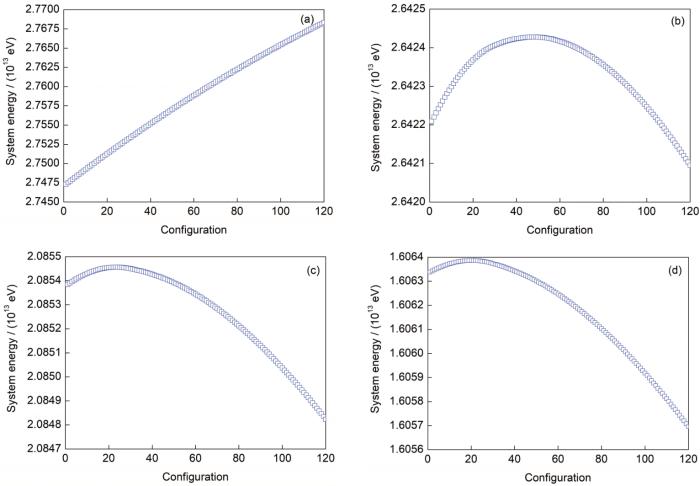
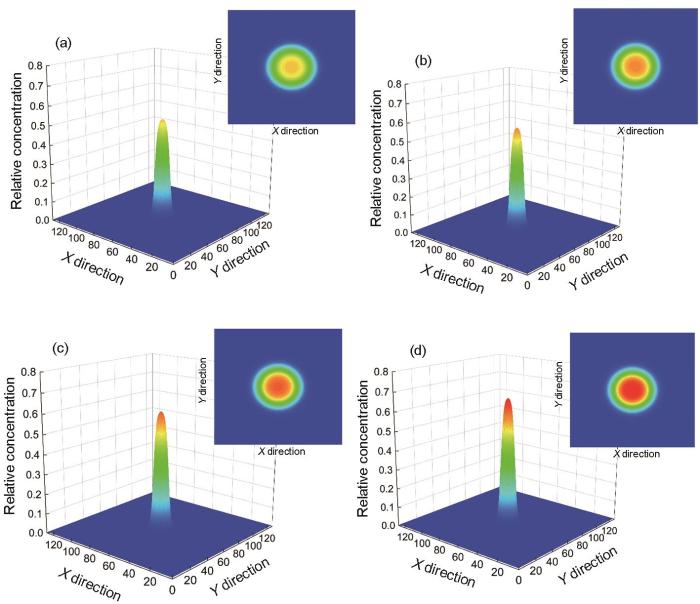
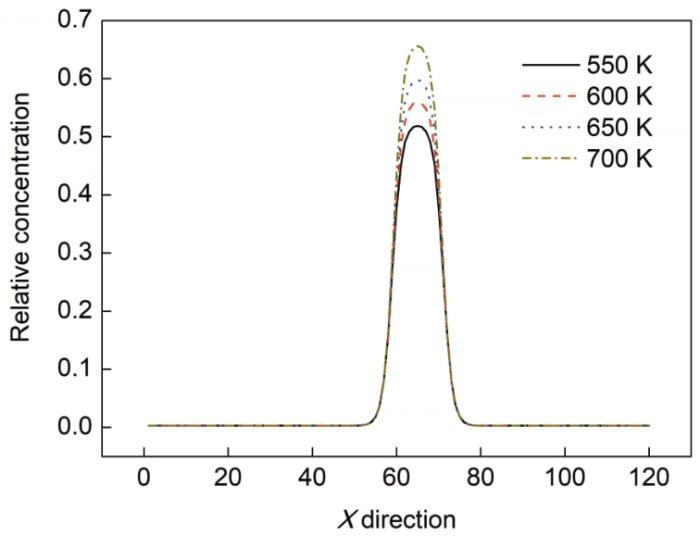
图4为不同温度下演化50步(I = 50)后Fe-0.3%Cu合金中富Cu相形核的最小能量路径。可以看出,温度对能量路径的影响非常明显。当温度为550 K时(图4a),在模拟时间内没有观察到能量路径的峰值点,而当温度高于600 K时(图4b~d),在模拟时间内观察到了明显的能量路径的峰值。这说明随温度降低形核速率在大幅延缓,形核所需时间大幅延长,表明温度的降低将大大增加形核难度。李博岩[21]的计算结果显示,当温度降低到一定程度,形核势垒将迅速升高到常规热力学手段难以达到的高度,这与本工作计算结果类似。这说明在辐照条件下温度将极大影响形核过程的方向,即Fe-Cu二元合金的富Cu相析出过程具有明显的温度驱动性。
图4
图4
不同温度下演化50步后Fe-0.3%Cu合金的最小能量路径
Fig.4
Minimum energy path curves of Fe-0.3%Cu alloy after 50-step-revolution at 550 K (a), 600 K (b), 650 K (c), and 700 K (d)
图5
图5
不同温度下演化50步后Fe-0.3%Cu合金临界团簇的Cu元素浓度分布和团簇形貌
Fig.5
Cu concentration distributions and top views of cluster morphologies of critical nucleus (insets) of Fe-0.3%Cu alloy after 50-step-revolution at 550 K (a), 600 K (b), 650 K (c), and 700 K (d)
表4 不同温度下演化50步后Fe-0.3%Cu合金临界团簇半径与浓度计算结果
Table 4
| T / K | RC / nm | CC / % |
|---|---|---|
| 550 | 1.722 | 45.4355 |
| 600 | 1.762 | 47.8542 |
| 650 | 1.794 | 50.2122 |
| 700 | 1.821 | 52.5147 |
图6
图6
不同温度下演化50步后Fe-0.3%Cu合金临界团簇Cu元素浓度的一维分布曲线
Fig.6
1D Cu concentration distribution curves in critical nucleus cluster of Fe-0.3%Cu alloy after 50-step-revolution at different temperatures
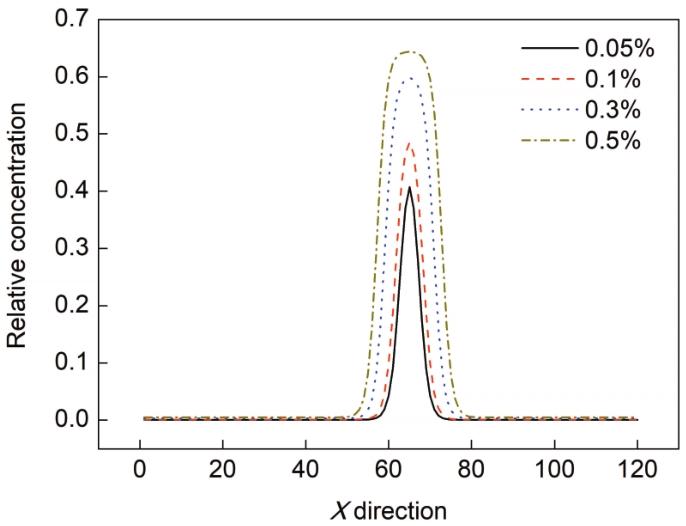
2.3 Cu含量对形核的影响
图7
图7
650 K下演化50步后不同Cu元素浓度Fe-Cu合金的最小能量路径
Fig.7
Minimum energy path curves of Fe-Cu alloy after 50-step-revolution with Cu concentrations of 0.05% (a), 0.1% (b), 0.3% (c), and 0.5% (d) at 650 K
图8
图8
650 K下演化50步后不同Cu元素元素浓度Fe-Cu合金临界团簇的Cu元素浓度分布和团簇形貌
Fig.8
Cu concentration distributions and top views of cluster morphologies of critical nucleus (insets) of Fe-Cu alloy after 50-step-revolution with Cu concentrations of 0.05% (a), 0.1% (b), 0.3% (c), and 0.5% (d) at 650 K
表5 650 K下演化50步后不同Fe-Cu合金的临界团簇半径与平均浓度计算结果
Table 5
| Alloy | RC / nm | CC / % |
|---|---|---|
| Fe-0.05Cu | 1.190 | 26.9862 |
| Fe-0.1Cu | 1.292 | 35.3291 |
| Fe-0.3Cu | 1.762 | 47.8542 |
| Fe-0.5Cu | 2.014 | 52.8991 |
图9
图9
650 K下演化50步后不同Fe-Cu合金临界团簇中Cu元素浓度的一维分布曲线
Fig.9
1D Cu concentration distribution curves in critical nucleus clusters of Fe-Cu alloy with different Cu concentrations at 650 K after 50-step-revolution
图10
图10
不同温度和不同Cu元素浓度下演化50步后团簇半径的变化曲线
Fig.10
Changes of cluster radius for Fe-Cu alloy after 50-step-revolution
(a) 650 K for different Cu concentrations
(b) 0.3%Cu concentration at different temperatures
2.4 辐照剂量对空位产生与分布的影响
辐照空位的产生与材料成分、辐照强度有关,而与温度关系不明显。在材料中存在空位产生、空位与间隙原子复合、空位被晶界或其他缺陷吸收3种效应。
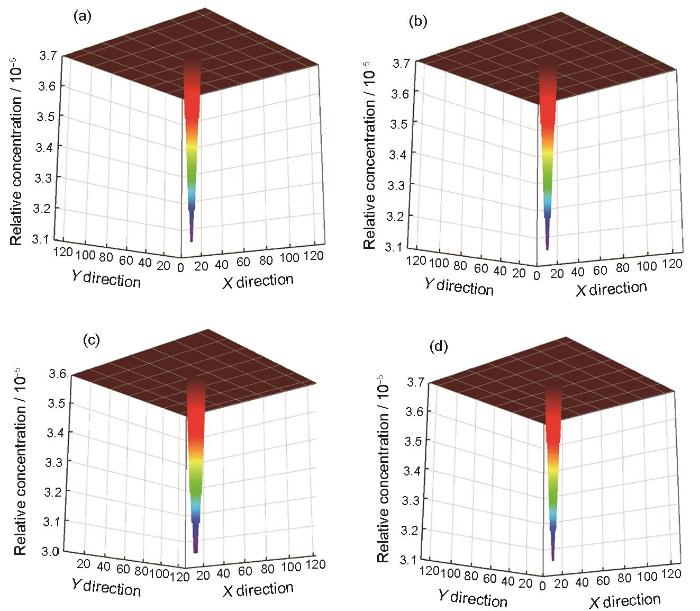
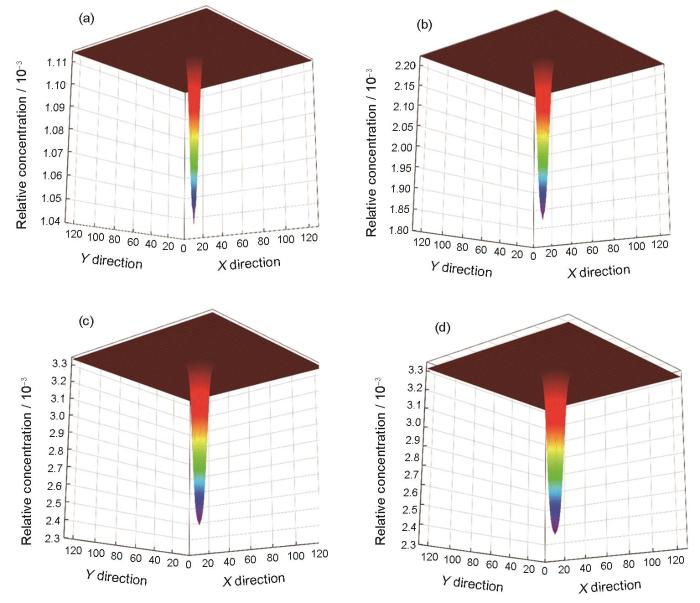
图11为650 K下演化50步后在辐照强度分别为0.01和0.05 dpa/s下Fe-0.3%Cu和Fe-0.5%Cu合金在临界团簇状态下的空位增量分布图。其中0.01 dpa/s时Fe-0.5%Cu合金富Cu团簇中的空位相对浓度增加量最少,为3.006 × 10-5;0.05 dpa/s时Fe-0.3%Cu合金富Cu团簇的空位相对浓度增加量最多,为3.15 × 10-5。可以看出,与基体中空位浓度的增加值相比,富Cu团簇中的辐照空位浓度增加量较少。可见,辐照条件下空位浓度的变化受Cu含量的影响较大。而提高辐照强度后,基体和富Cu团簇中的空位浓度均有所提高。
图11
图11
650 K下演化50步后不同辐照强度下Fe-0.3%Cu和Fe-0.5%Cu合金临界团簇状态的空位增量分布图
Fig.11
Distributions of the increase of vacancy concentration at critical nucleus cluster state after 50-step-revolution at 650 K
(a) Fe-0.3%Cu, 0.01 dpa/s (b) Fe-0.3%Cu, 0.05 dpa/s
(c) Fe-0.5%Cu, 0.01 dpa/s (d) Fe-0.5%Cu, 0.05 dpa/s
图12
图12
650 K下演化50步后辐照强度0.01 dpa/s下Fe-0.3%Cu合金在不同时刻的空位浓度分布
Fig.12
Vacancy concentration distributions after 50-step-revolution at the different time (t) of nucleus of Fe-0.3%Cu alloy at 650 K and 0.01 dpa/s
(a) t = 30 step (b) t = 60 step (c) t = 90 step (d) t = 120 step
表6 演化50步后不同时刻下团簇空位浓度信息计算结果
Table 6
| t / step | RC / nm | Min.CV | Max.CV - Min.CV | Sum.CV |
|---|---|---|---|---|
| 30 | 1.558 | 0.00102 | 8.63 × 10-5 | 17.149 |
| 60 | 1.763 | 0.00182 | 3.89 × 10-4 | 34.381 |
| 90 | 1.884 | 0.00229 | 8.92 × 10-4 | 51.535 |
| 120 | 2.039 | 0.00280 | 1.65 × 10-3 | 69.190 |
3 结论
(1) 建立了结合辐照空位形成的Fe-Cu二元合金Cu元素析出的相场模型,结合约束条件弦方法对辐照条件下Fe-Cu合金中富Cu相形核过程进行了计算,得到了在多种温度和初始浓度下Cu元素形核过程临界形核状态的纳米尺寸Cu元素团簇物理参数、形核过程能量路径、空位分布等参数,计算结果与实验结果基本符合。
(2) 温度和Cu含量对Fe-Cu二元合金中富Cu相析出过程的能量路径和临界形核尺寸有明显影响。其中,温度是影响形核能量路径走向的主要因素,浓度是影响临界形核半径生长速率的主要因素。温度越高,Cu含量越高,形核达到临界形核尺寸所需要的时间越短,所需要超越的能量势垒越低,与实验观察结果一致。
(3) Cu元素浓度场的分布对辐照空位浓度的分布也有很大的影响。Cu元素含量越高,相同辐照条件下空位浓度越低,且空位的分布越不均匀。在富Cu团簇中的空位浓度比Fe-Cu基体更低,而且Cu浓度越高,空位的浓度越低。
参考文献
Irradiation embrittlement mechanisms and relevant influence factors of nuclear reactor pressure vessel steels
[J].
核能系统压力容器辐照脆化机制及其影响因素
[J].核反应堆压力容器作为核电站不可更换的关键性设备, 其设备完整性对核电站的安全运行起着至关重要的作用. 在辐照条件下, 反应堆压力容器钢中会形成一系列微结构缺陷, 包括溶质沉淀、基体损伤和脆性元素的晶界偏聚等, 导致材料的韧脆性转变温度升高, 产生辐照脆化效应. 而压力容器钢的成分和辐照条件决定了各种微结构对辐照脆化的贡献大小. 本文主要针对核能系统压力容器辐照脆化机制及其影响因素进行了综述, 总结讨论了这些微结构的形成机制及溶质元素、辐照通量和辐照后退火对这些微结构和材料机械性能的影响, 并指出了存在的问题和未来的研究方向.
The 885°F (475oC) embrittlement of ferritic stainless steels
[J].
Detection of intergranular embrittlement of reactor pressure vessel steel by electrochemical method
[J].
Contributions of Cu-rich clusters, dislocation loops and nanovoids to the irradiation-induced hardening of Cu-bearing low-Ni reactor pressure vessel steels
[J].
Influence of different chemical elements on irradiation-induced hardening embrittlement of RPV steels
[J].
Transmission electron microscopy study on neutron irradiated pure iron and RPV model alloys
[J].
Thermodynamic and kinetic modeling of Mn-Ni-Si precipitates in low-Cu reactor pressure vessel steels
[J].
Phase field simulations of the sintering process of UO2
[J].
UO2烧结过程的相场模拟
[J].利用相场模型对UO<sub>2</sub>陶瓷粉末的烧结过程进行了模拟。在修正的相场模型中,不仅考虑了表面扩散、晶界扩散和晶格扩散3种各向异性的扩散机制对烧结组织形貌和烧结动力学的影响,而且考虑了不同陶瓷颗粒之间的界面能对烧结形貌的影响。基于实验条件和热力学物性参数,对UO<sub>2</sub>陶瓷粉末在2000 K的烧结过程进行了模拟。模拟结果显示:初始形貌为圆形的陶瓷粉末有利于烧结过程的进行;烧结过程中存在大晶粒吞噬小晶粒的现象;晶界扩散机制是UO<sub>2</sub>烧结过程中的主导机制;晶界能的改变导致晶界与相界之间的平衡二面角发生改变。在此基础上,模拟了多晶UO<sub>2</sub>陶瓷粉末的烧结过程,模拟结果与实验结果吻合较好。
Phase-field modeling of pores and precipitates in polycrystalline system
[J].
Phase-field simulation of solid state sintering
[J].
Computer simulation of grain growth using a continuum field model
[J].
Multiscale simulations on the coarsening of Cu-rich precipitates in α-Fe using kinetic Monte Carlo, molecular dynamics and phase-field simulations
[J].
Phase field study of the copper precipitation in Fe-Cu alloy
[J].
Effect of Mn composition on the nanometer Cu-rich phase of Fe-Cu-Mn alloy by phase field method
[J].
Mn含量对Fe-Cu-Mn合金纳米富Cu析出相影响的相场法研究
[J].采用相场法模拟了Fe-Cu-Mn合金在823 K时效时富Cu析出相的三维组织演化图像、体积分数、数量密度和平均颗粒半径随时效时间的变化等。相场模拟研究表明:相分离早期阶段通过失稳分解机制形成富Cu相;同时Mn原子也在富Cu相的中心发生偏聚,在富Cu相开始Ostwald粗化的过程中,Mn原子又从其核心处偏聚到富Cu相和基体的界面处,最终在富Cu相外部形成富Mn环。富Mn环的存在会抑制富Cu相的扩散长大和粗化;富Cu相在时效前期是球状的bcc结构,随着不断长大,转变为椭球形或棒状的fcc结构;提高Mn含量可以加快富Cu相的析出,有利于富Cu相的粗化。
Vacancy and interstitial atom evolution with the separation of the nanoscale phase in Fe-Cr alloys: Phase-field simulations
[J].
Simulations of irradiated-enhanced segregation and phase separation in Fe-Cu-Mn alloys
[J].
Simplified and improved string method for computing the minimum energy paths in barrier-crossing events
[J].
String method in collective variables: Minimum free energy paths and isocommittor surfaces
[J].
Modelling and simulation in radiation induced defects in reactor pressure vessel steels
[D].
反应堆压力容器钢辐照损伤的计算模拟研究
[D].
Finding Non-classical critical nuclei and minimum energy path of Cu precipitates in Fe-Cu alloys
[J].
Mathematical and numerical aspects of a phase-field approach to critical nuclei morphology in solids
[J].
Diffuse-interface approach to predicting morphologies of critical nucleus and equilibrium structure for cubic to tetragonal transformations
[J].
Applications of semi-implicit Fourier-spectral method to phase field equations
[J].
Computer modeling and simulation of solid-state sintering: A phase field approach
[J].
Free energy of a nonuniform system. III. Nucleation in a two-component incompressible fluid
[J].
A phase-field model for evolving microstructures with strong elastic inhomogeneity
[J].
Research on atomic states, physical properties and catalytic performance of Ru metal
[J].
Migration of Cu adatoms on a Cu(100) surface, studied with low-energy ion scattering (LEIS)
[J].
Flux effects in precipitation under irradiation—Simulation of Fe-Cr alloys
[J].
Irradiation hardening in copper and nickel
[J].
On the effect of dose rate on irradiation hardening of RPV steels
[J].
Effect of Cr-rich phase precipitation on magnetic and mechanical properties of Fe-20% Cr alloy
[J].
Simulation of the growth of copper critical nucleus in dilute bcc Fe-Cu alloys
[J].
On asymptotic limits of Cahn-Hilliard systems with elastic misfit
[A].
Computer simulation of phase decomposition in Fe-Cu-Mn-Ni quaternary alloy based on the phase-field method
[J].
Non-classical nuclei and growth kinetics of Cr precipitates in FeCr alloys during ageing
[J].
Cu precipitation in a prestrained Fe-1.5 wt pct Cu alloy during isothermal aging
[J].
Optimization of the critical nuclear size for protein crystallization: A note
[J].