残余应力是材料内部不同区域不协调导致的内应力。从尺度方面可以将其分为3类:第Ⅰ类内应力(σⅠ) 是较大体积内宏观尺度不协调导致的,也称为宏观应力;几个晶粒尺度或不同相之间的应力通常被称为第Ⅱ类内应力(σⅡ),是一种微观应力;第Ⅲ类内应力(σⅢ)尺度更小,一般将原子尺度上不均匀行为产生的应力称为σⅢ,通常来自于位错、元素掺杂或辐照引起的晶格畸变等。充分退火对于消除金属材料宏观应力效果明显,并且已经得到广泛应用。对于复合材料,为了获得更好的去应力效果,时效和热冷循环被广泛使用。热冷循环是将材料循环置于时效温度和低温环境中进行保温,以降低应力的处理方式。Qu等[10]的研究表明,热冷循环的应力降低效果优于时效去应力,并且热冷循环处理6 cyc已将应力降低到较低水平。热冷循环在时效温度时基体会产生析出相,时效温度到低温转变时会产生热冲击,热冲击会使基体在界面附近产生位错倍增[11]。析出相和位错增多时会伴随微塑性变形的发生,因此时效和热冷循环处理的应力降低机制都可以归结为微塑性变形的发生[10]。
本工作使用中子衍射和有限元模拟相结合的方法,研究深冷循环次数和深冷循环温度对不同尺寸铝基复合材料宏微观应力的影响。制备了2种不同颗粒尺寸的35%SiC/6092Al (体积分数,下同)复合材料,并且制备了2种尺寸的圆饼状试样,研究颗粒尺寸和样品尺寸对深冷循环后残余应力的影响,分析深冷循环次数、温度差、增强相尺寸、样品尺寸对宏微观应力的影响机制,旨在为铝基复合材料残余应力的调控提供数据基础和理论指导。
1 实验方法
1.1 材料制备及表征
制备了SiC粉粒径分别为7和14 μm、体积分数为35%的2种SiC/6092Al复合材料。6092Al基体合金的名义成分为Al-1Mg-1Si-0.85Cu (质量分数,%),实验采用的金属粉末和SiC粉末纯度都在99.5%以上。材料制备选择机械混合后的粉末进行真空热压烧结,获得热压态块体材料。将每种块体材料机加工成直径50 mm、厚10 mm (D50)和直径120 mm、厚30 mm (D120) 2种圆饼状试样并进行退火处理。退火制度为450℃保温4 h,随炉冷却,冷却时长24 h。使用Apreo2c扫描电子显微镜(SEM)观察颗粒分布,试样表面研磨到3000号砂纸并进行机械抛光。
不同深冷循环次数的对比实验均选用尺寸为7 μm SiC颗粒的D120退火态试样。深冷循环温度为100~-196℃,深冷循环次数分别为1、3、6 cyc。深冷循环过程中100℃保温1 h,-196℃保温30 min,以保证深冷循环过程中试样整体冷热充分。不同深冷循环温度差实验选用100~-196℃和200~-196℃ 2个深冷循环温度,试样尺寸为D50。
1.2 中子衍射应力测量
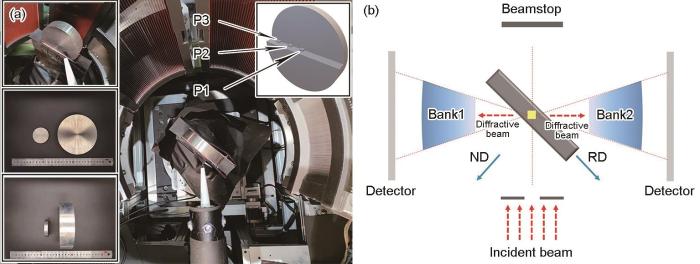
使用中国散裂中子源(CSNS)的工程材料中子衍射仪(EMD)测量D120退火态试样的应力,测量使用波长范围0~0.3 nm,取样体积6 mm × 6 mm × 6 mm,单点测量时长1 h。其余试样使用CSNS通用粉末衍射仪(GPPD)[23]进行应力测量(GPPD在2020年进行了升级改造,目前具备材料结构和残余应力测定的能力[24,25]),测试使用波长范围0.05~0.4 nm,取样体积为直径5 mm、厚2 mm。厚度10 mm试样测试时间为2 h,厚度30 mm试样测试时间为3 h。为保证测量结果准确,GPPD应力测量试样均测量了3个位置,分别是中心点(P1),沿中心半径的1/3点(P2)和2/3点(P3),下文中未加说明的点均为中心点应力结果对比。相应力选取Al(311)晶面和SiC(116)晶面进行计算,并以此计算宏观应力[20]。应力测量选用SiC粉末作为SiC相的无应力试样(D0试样),Al相的D0试样使用对应基体合金碎屑。试样摆放、测试位置点剖视图及衍射光路如图1所示,其中ND、RD和TD分别表示圆饼状试样的轴向、径向和周向。将D0试样放入直径9 mm的钛锆合金样品盒进行测试,所有测试在同一衍射光路下进行,测量期间不改变入射狭缝、径向准直器和探测器位置。
图1
图1
中子衍射应力测现场图及尺寸图,及中子衍射光路图
Fig.1
Neutron diffraction stress measurement site graphic and dimensional graphic (a), and neutron diffraction light path diagram (b) (ND—normal direction, RD—radial direction)
Al和SiC相应力(σAl和σSiC)通过弹性应变(εhkl )计算获得,在飞行时间(TOF)中子衍射测量模式下,弹性应变的计算公式[26]为:
式中,d0,hkl 为D0试样的晶面间距;Δdhkl 为应力导致的晶面间距变化值;t0,hkl 为D0样品的飞行时间;thkl 为测量样品的飞行时间。
通过晶面间距计算的应力结果为Al和SiC的相应力,相应力与宏微观应力的关系如下式[27]:
式中,σx 为x (x为Al或SiC)的相应力;f为增强相SiC的体积分数;
1.3 数值模拟方法
图2
图2
35%SiC/Al复合材料代表性体积单元模型
Fig.2
Representative volume element of 35%SiC/Al composites
(a) reinforcement particles (b) matrix and reinforcement particles
表1 数值模拟中使用的材料参数
Table 1
| Phase | Parameter | Value | Unit |
|---|---|---|---|
| Al | Conductivity | 174.57[30] | mJ·(s·mm·oC)-1 |
| Young's modulus | 68.00 | GPa | |
| Poisson's ratio | 0.33 | - | |
| Thermal expansion coefficient | 2.30 × 10-5 | K-1 | |
| Yield stress | 200 | MPa | |
| SiC | Conductivity | 120.00 | mJ·(s·mm·℃)-1 |
| Young's modulus | 415.00 | GPa | |
| Poisson's ratio | 0.17 | - |
因为高温下基体材料的强度低,所以模拟假设高温下RVE模型处于无应力状态。为了获得材料退火后初始的应力状态,先进行从450℃高温到25℃室温的退火过程模拟,获得退火态后的初始应力状态。深冷循环过程模拟100~-196℃的冷热循环过程,循环次数为6 cyc,最终模型温度为25℃,与中子衍射应力测试时的温度状态一致。整个RVE模型的6个面均为自由面,为了避免模型的刚体位移,在底面相交的2条棱上添加了仅能沿边方向移动的位移约束条件。
2 实验结果与讨论
2.1 不同颗粒尺寸初始退火态试样状态
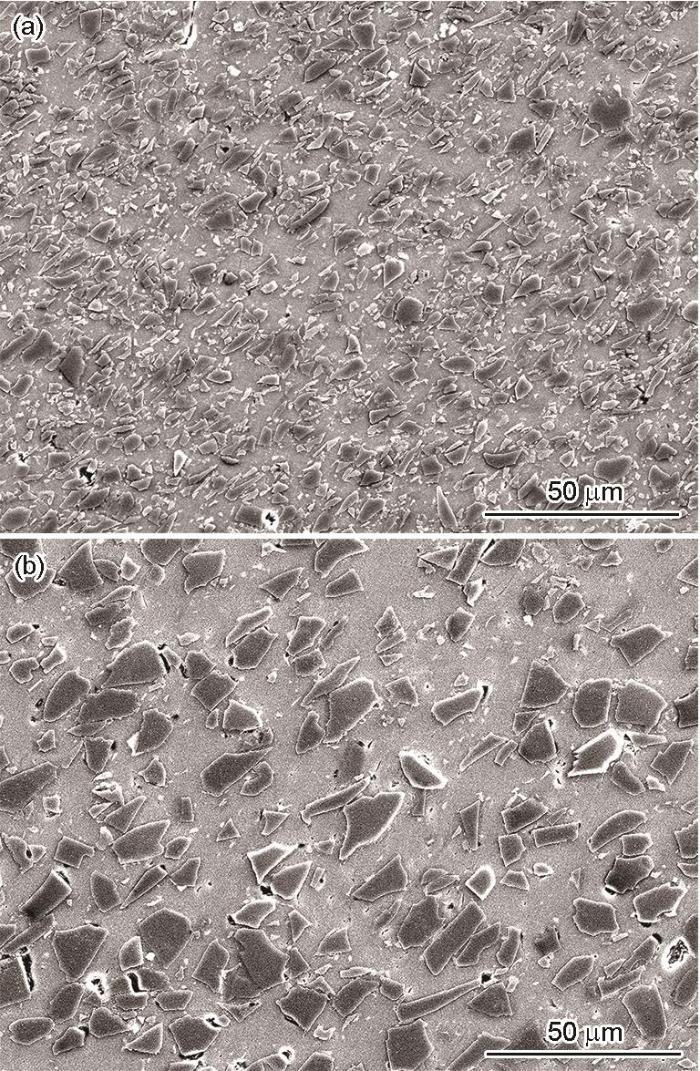
图3
图3
不同SiC颗粒尺寸初始退火态SiC/6092Al复合材料的SEM像
Fig.3
SEM images of initial annealed SiC/6092Al composites with the SiC particle sizes of 7 μm (a) and 14 μm (b)
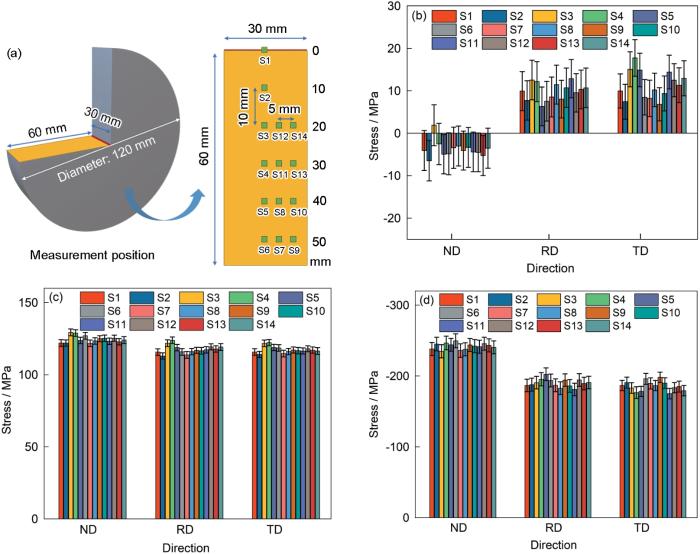
为了与深冷循环后复合材料的残余应力进行对比,使用中子衍射应力分析表征退火态复合材料的宏微观应力及其分布。图4a和b为7 μm SiC颗粒的D120退火态试样应力测量位置和结果。可明显看出,SiC/6092Al复合材料退火后不同位置应力差别均在误差范围内。材料ND方向宏观应力为负,RD和TD方向宏观应力为正,但3个方向宏观应力都接近于0 MPa,并且最大值低于20 MPa (20 MPa已经低于中子衍射应力测量精度范围)。因此,可以说明D120的退火态圆饼状SiC/6092Al复合材料试样的宏观应力接近于0 MPa。14 μm SiC颗粒增强的SiC/6092Al退火态试样宏观应力也接近于0 MPa,具体结果在2.4节给出。
图4
图4
试样尺寸和应力测量位置,及宏观应力、Al相应力、SiC相应力结果
Fig.4
Sample size and stress measurement locations S1-S14 (a), and results of macroscopic stress (b), Al phase stress (c), and SiC phase stress (d)
2.2 深冷循环应力降低效果
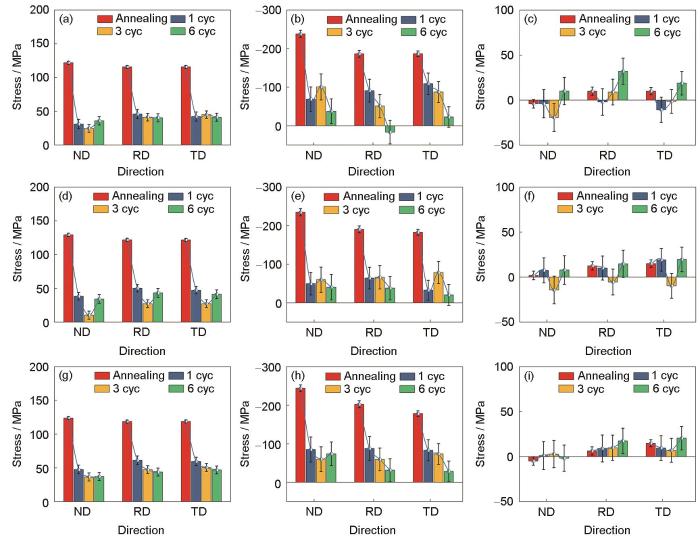
直径120 mm、厚30 mm的退火态试样(下文称D120试样)深冷循环次数对宏微观应力的影响如图5所示。图5a~c为D120试样P1应力测量结果。由图5a和b可见,深冷循环1 cyc就能明显降低Al基体和增强相SiC的相应力,并且其宏观应力变化相对较小(图5c)。随着循环次数的增加,Al相应力变化较小,SiC相应力具有明显降低趋势,当循环次数为6 cyc时,SiC相应力基本接近于0 MPa。P1、P2和P3规律相近,首次深冷循环Al和SiC相应力降低最明显,但随深冷循环次数增加,相应力变化不明显。而P1、P2和P3的宏观应力在ND、RD、TD方向都接近于0 MPa,仅在深冷循环6 cyc时的RD和TD有明显大于0 MPa的拉应力。可见,随深冷循环次数的增加,Al和SiC微观相应力均不断降低,且SiC相应力降低更明显。
图5
图5
D120试样不同深冷循环次数时Al和SiC相应力及材料宏观应力对比
Fig.5
Al internal stresses (a, d, g), SiC internal stresses (b, e, h), and macroscopic stresses (c, f, i) of D120 sample in different cryogenic cycles (a-c) P1 (d-f) P2 (g-i) P3
图6
图6
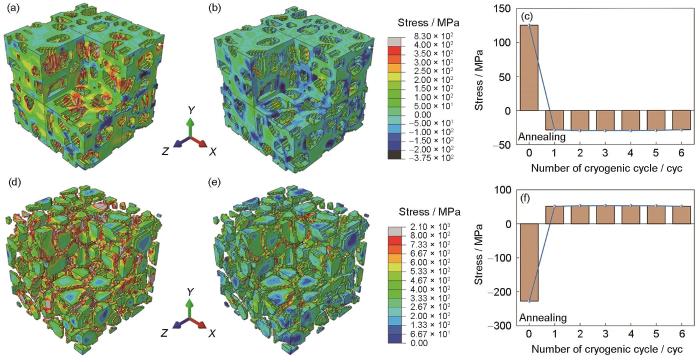
D120试样深冷循环前后Al和SiC的相应力
Fig.6
Internal stresses of Al phase (a-c) and SiC phase (d-f) of D120 sample before and after cryogenic cycles
(a, d) annealed stress distributions
(b, e) stress distributions of 1 cryogenic cyc
(c, f) average stress diagrams of different cryogenic cycles
图7
图7
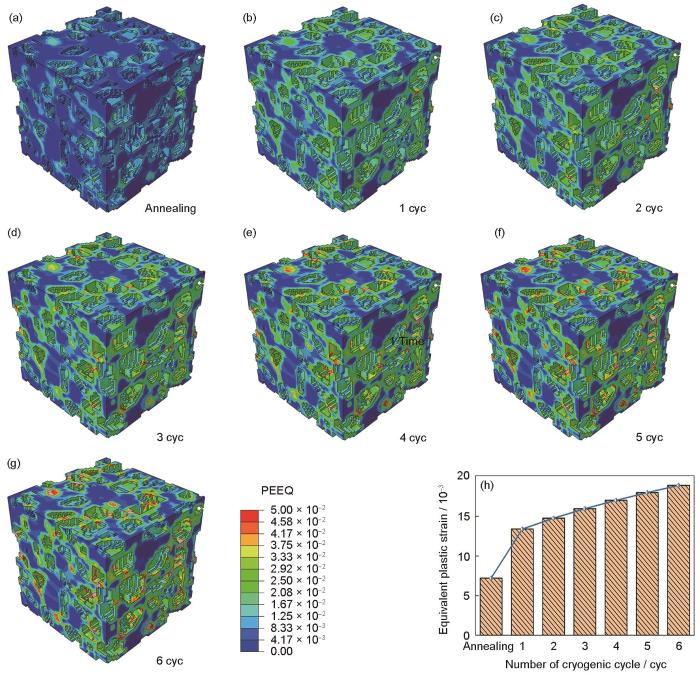
D120试样不同深冷循环后Al相等效塑性应变的分布情况及基体等效塑性应变对比图
Fig.7
Distributions of equivalent plastic strains (PEEQ) in Al phase after different cryogenic cycles of D120 sample
(a-g) annealed state (a) and 1-6 cryogenic cyc, respectively (b-g)
(h) comparison of equivalent plastic strains in matrix with different number of cryogenic cycles
2.3 样品尺寸对材料宏微观应力影响
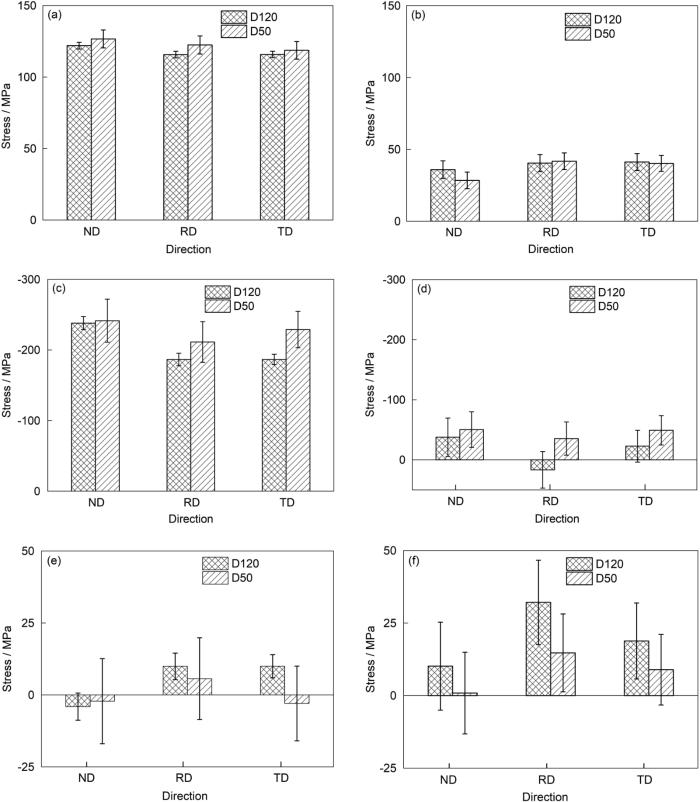
一般认为宏观残余应力受到样品尺寸的影响[39],而样品尺寸对微观残余应力影响却缺乏研究。本工作使用了D120和D50 2种尺寸的样品,2种样品均为SiC颗粒标称粒径为7 μm的SiC/6092Al复合材料。使用中子衍射应力分析对比研究了退火态和深冷循环6 cyc (100~-196℃)后的微观应力,结果如图8所示。其中,图8a、c和e为退火态试样应力结果,图8b、d和f为深冷循环6 cyc后的应力结果。由图8a可见,D120和D50 2种尺寸样品退火处理后Al相应力基本一致,都在120 MPa左右。且ND、RD、TD 3个方向上应力也基本一致。对比图8c发现,ND方向D120和D50 2种样品应力基本一致,而RD和TD方向D120样品的应力较D50样品小约20 MPa,且2种误差棒有重叠。图8e中D120和D50样品的宏观应力也基本为0 MPa。说明2种材料退火后,D120和D50样品宏观应力基本为0 MPa,且Al和SiC相应力在ND、RD、TD 3个方向上相差不大。
图8
图8
不同尺寸的样品深冷循环前后相应力和宏观应力对比图
Fig.8
Al internal stresses (a, b), SiC internal stresses (c, d), and macroscopic stresses (e, f) in different sample sizes at annealed state (a, c, e) and 6 cryogenic cyc (b, d, f)
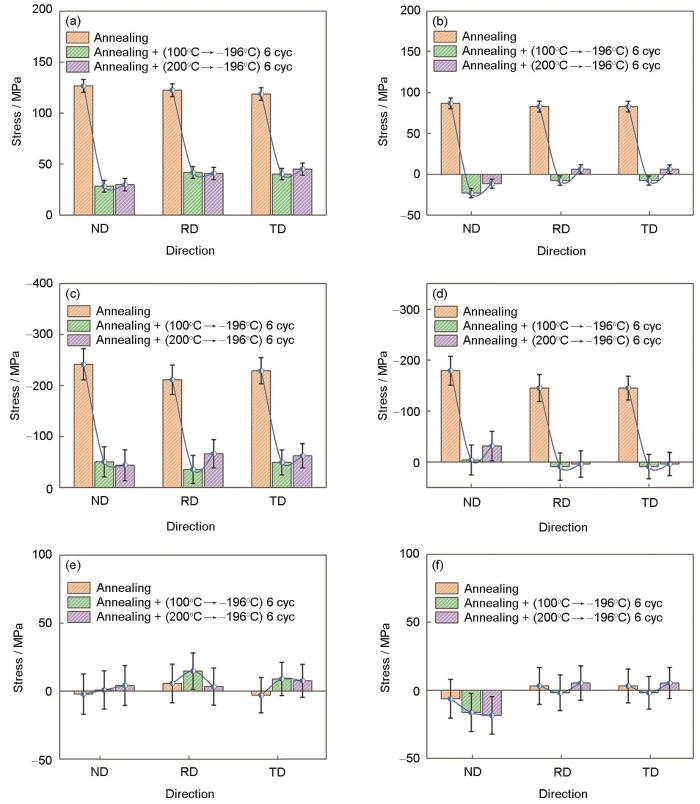
2.4 深冷循环温度差对应力的影响
一般认为深冷循环的温度差对相应力的降低影响较大[40],因此选取了100~-196℃和200~-196℃ 2个深冷循环温度差进行应力对比。同时,为了研究增强相尺寸对复合材料应力的影响,选取了7和14 μm SiC颗粒增强的SiC/6092Al进行宏微观应力对比,结果如图9所示。图9a和b显示,相较于退火态,2种不同温度差的深冷循环6 cyc后,Al相应力在ND、RD、TD 3个方向上都明显降低,但100~-196℃和200~-196℃ 2种深冷循环温度差处理后的7 μm SiC颗粒增强复合材料的Al相应力基本一致(图 9a),而14 μm SiC颗粒增强复合材料中100~-196℃温度差比200~-196℃温度差处理后的Al相应力低10 MPa左右。图9c和d中SiC相应力在深冷循环后明显降低,但100~-196℃和200~-196℃ 2种温度差的深冷循环处理后SiC相应力基本相同。图9e和f中100~-196℃和200~-196℃ 2种温度差的深冷循环处理后的宏观应力也基本相同。
图9
图9
不同SiC颗粒尺寸在不同深冷循环温度差下相应力和宏观应力
Fig.9
Al internal stresses (a, b), SiC internal stresses (c, d), and macroscopic stresses (e, f) in different SiC particle sizes at 7 μm SiC (a, c, e) and 14 μm SiC (b, d, f)
对比7和14 μm 2种SiC颗粒增强的复合材料深冷循环前后的应力结果可得,深冷循环6 cyc后,Al相应力都降低了100 MPa左右。7 μm增强退火态SiC/6092Al复合材料的Al相应力高于14 μm颗粒增强的退火态材料的Al相应力(图9a和b)。100~-196℃深冷循环6 cyc后Al和SiC相应力降低值比200℃~-196℃高10~20 MPa。在图9c和d的SiC相中也呈现出了相似规律。同时,图9a和b中的Al相应力在ND、RD、TD 3个方向上分布不相同,RD和TD的应力比ND方向高 20 MPa左右。从图9e和f能够看出,2种颗粒尺寸和2个深冷循环温度差导致材料宏观应力的变化并不明显,基本都在0 MPa附近。
以上结果说明多次深冷循环后铝基复合材料的相应力能够降低到一个相对低的水平,深冷循环的温度差对材料宏微观应力的影响不大。不同SiC颗粒尺寸的复合材料在相同温度差的深冷循环后降低的相应力基本一致。这说明多次深冷循环处理降低应力的效果与增强相颗粒尺寸无关。
3 结论
(1) 深冷循环能够降低SiC/6092Al复合材料中Al和SiC相应力,深冷循环1 cyc降低效果最明显,随深冷循环次数增加,Al和SiC相应力继续降低,但深冷循环降低相应力的效果减弱,且深冷循环不会增加退火态样品的宏观应力。深冷循环降低相应力是由于基体发生塑性应变导致的,并且随着深冷循环次数的增加,基体的位错密度会明显上升。
(2) 深冷循环对SiC/6092Al复合材料相应力的降低与其样品尺寸无关。完全退火处理后样品宏观应力接近于0 MPa,深冷循环影响的是Al和SiC微观相应力,微观相应力是数个晶粒或Al和SiC之间的应力,其变化与材料样品尺寸无关。
(3) 多次深冷循环后,深冷循环温度差对相应力的降低影响较小。这是由于多次深冷循环后应力已经通过基体塑性变形降低到一个较低水平,多次深冷循环逐渐消除了深冷循环温度差的影响。且多次深冷循环后对不同SiC颗粒尺寸材料的相应力降低效果基本相同。
参考文献
Metal matrix composites—From science to technological significance
[J].
Research and development of medium/high volume fraction SiCp/Al composites
[J].
中高体积分数SiCp/Al复合材料研究进展
[J].
Metal-matrix composites for space applications
[J].
Recent progress of reinforcement materials: A comprehensive overview of composite materials
[J].
Residual stress. Part 2—Nature and origins
[J].
Residual stress and its role in failure
[J].
Research progress on principle of dimensional stability and stabilization design of Al and its composites
[J].Dimensional stability refers to the materials' ability to maintain their original size during long-term storage or under service conditions. The key components of the angle, velocity and position sensors, such as the gyroscopes, star sensors and optical observation devices, are extreme sensitive to the micro-deformation of the materials, and the dimensional instability of the present materials has become to be the bottleneck problem that restricts the accuracy of the equipment. Deep research has been carried out abroad from the aspect of the microstructure modification by heat-treatment and pretension deformation treatment of metals since 1970s. However, the domestic research on the dimensional stability is rather weak, which was mainly focused on the effect of the residual stress, and the corresponding engineering application effect is not pronounced. In the present work, the research experience and main results of the authors and coworkers on dimensional stability for decades have been introduced, including novel characterization method of dimensional stability during long-term storage (without stress), and the basic evolution process of the phase-stability, microstructure-stability and the anisotropy behavior of the Al alloys, which was revealed by the novel characterization method. Furthermore, the basic design principles of high dimensional stability and the design ideas based on the dispersivity of reinforcements of the Al matrix composites have been introduced. Moreover, the microstructure characters and the application effect in practical engineering of the high dimensional stability optical-grade and instrument-grade SiC/2024Al composites have been described. Based on the theoretical analysis and the practice effects, it indicates that the accuracy and accuracy stability of the instruments is mainly depended on dimensional stability of the used materials, and the dimensional stability of the materials was mainly affected by its intrinsic deformation characteristics, while the effect of the residual stress was subordinate. The present work also indicates that the application of the dimensional stability principle is also instructive to the technology upgrading of the high precision components, such as precision bearings.
Al及其复合材料尺寸稳定性原理与稳定化设计研究进展
[J].尺寸稳定性是指材料在长期贮存或者服役环境下保持原始尺寸不变的能力。陀螺仪、星敏感器、光学观瞄设备等角度、速度、位置传感器的关键零部件对材料的微变形十分敏感,材料的尺寸不稳定性问题已经成为制约装备精度的“卡脖子”问题。国外自20世纪70年代从金属的热处理、预拉伸变形等组织调控方法入手做了较深入的研究,我国关于材料尺寸稳定性的研究十分薄弱,主要集中于残余应力的影响上,工程效果不明显。本文介绍作者及其团队长期从事材料尺寸稳定性研究的体会与成果,包括长期贮存(无应力)条件下尺寸稳定性的表征新方法,基于该方法发现了铝合金相稳定、组织稳定及其各向异性的基本规律;总结了铝基复合材料尺寸稳定性设计的基本原理和基于增强体弥散度的设计思路;高尺寸稳定性的光学级、仪表级SiC/2024Al复合材料的微观构型特征及其在实际工程中应用的效果。理论和实践表明,仪表精度及其精度稳定性取决于材料的尺寸稳定性,而材料的稳定性首要因素是其内禀变形特性,而残余应力是次要的。本工作也表明,尺寸稳定性原理的应用对于精密轴承一类高精度零件的技术提升也将有启发性。
Microstructural evolution and dimensional stability of 45vol% SiC/Al composites under long-term aging
[J].
Revealing the mechanism of internal stress on dimensional stability in SiC/Al composites under long-term thermal exposure
[J].
Effect of heat-treatment on stress relief and dimensional stability behavior of SiCp/Al composite with high SiC content
[J].
Effect of thermal shock induced dislocation multiplication on the thermal conductivity of 60 vol% SiCp/Al-7Si composites
[J].
Microstructure and improved plasticity of (FeCoNi1.5CrCu)p/Al composites subject to adjusted deep cryogenic treatment (DCT)
[J].
Effect of deep cryogenic treatment on microstructures and performances of aluminum alloys: A review
[J].
Explaining deviatoric residual stresses in aluminum matrix composites with complex microstructure
[J].
Residual stress. Part 1—Measurement techniques
[J].
Methods of measuring residual stresses in components
[J].
Mapping residual and internal stress in materials by neutron diffraction
[J].
Correlation between residual stresses and the strength differential effect in PM 6061Al-15 vol% SiCw composites: Experiments, models and predictions
[J].
Separation of macroscopic, elastic mismatch and thermal expansion misfit stresses in metal matrix composite quenched plates from neutron diffraction measurements
[J].
Determination of macroscopic and microscopic residual stresses in friction stir welded metal matrix composites via neutron diffraction
[J].
Microstructure-based analysis of residual stress concentration and plastic strain localization followed by fracture in metal-matrix composites
[J].
Thermo-mechanical analysis of uniform, clustered and interpenetrating phase particulate composites using finite element method
[J].
The general purpose powder diffractometer at CSNS
[J].
Multiscale residual stress evaluation of engineering materials/components based on neutron and synchrotron radiation technology
[J].Multiscale residual stress exists throughout the manufacturing process of engineering components, from design and production to processing and servicing. This stress can impact the machining accuracy, structural load capacity, and fatigue lifespan of these components. Therefore, accurate measurement and regulation of residual stress are critical for ensuring the longevity and reliability of engineering components. However, precise characterization of residual stress is challenging owing to its multilevel and cross-scale distribution traits and dynamic evolution under various conditions, such as temperature and load. Compared with laboratory X-ray measurement methods, neutron diffraction (ND), synchrotron-based high-energy X-ray diffraction (HE-XRD), and synchrotron-based X-ray microbeam diffraction (μ-XRD) techniques offer increased penetration depth and better time and spatial resolutions. In addition, the ability to attach environmental devices enables nondestructive and accurate in situ characterization of three types of residual stresses: macroscopic residual stress, intergranular or interphase microscopic stress, and intragranular ultramicroscopic stress. ND is currently the only nondestructive method capable of accurately measuring three-dimensional (3D) stress at centimeter-level depths within engineering components. HE-XRD, due to its high flux, excellent collimation, and millimeter-level penetration depth for metals, can be utilized for in situ studies of intergranular and interphase stress evolution and partitioning during deformation. The μ-XRD employs a submicron focused beam and differential aperture technology to analyze depth information of a sample. By conducting point-by-point scanning, it can capture 3D distribution of microscopic stress inside a single grain. Furthermore, our group has developed a novel method and device for depth stress characterization based on differential aperture technology under synchrotron-based high-energy monochromatic X-ray transmission geometry, and can measure stress gradients with high precision from the surface to the interior of engineering materials at millimeter-level depths. This study presents the measurement principles, application ranges, and applications of the above-mentioned multiscale stress characterization technologies based on the neutron/synchrotron facilities as well as envisaging the future development of related technologies.
基于中子与同步辐射技术的工程材料/部件多尺度残余应力评价
[J].多尺度残余应力贯穿于工程部件设计、生产、加工和服役的全生命周期,对工程部件的长寿命可靠服役具有重要意义。残余应力具有多层次、跨尺度的分布特征,在温度、载荷等服役环境作用下发生动态演化,给精确表征带来了很大困难。相较于传统实验室X射线残余应力测量方法,中子衍射、同步辐射高能X射线衍射和同步辐射微束衍射技术在穿透深度、时间分辨率、空间分辨率、环境装置等方面具有显著优势,能够实现宏观残余应力、晶间/相间微观应力、晶内超微观应力3类残余应力的原位无损精确表征。本文详细介绍了上述基于中子/同步辐射大科学装置的多尺度应力表征技术的测量原理、应用范围和典型应用案例,并对相关技术的发展进行了展望。
Residual stress measurement system of the general purpose powder diffractometer at CSNS
[J].
An enhanced finite element model considering multi strengthening and damage mechanisms in particle reinforced metal matrix composites
[J].
A fast numerical method of introducing the strengthening effect of residual stress and strain to tensile behavior of metal matrix composites
[J].Thermal residual stress and strain (TRSS) in particle reinforced metal matrix composites (PRMMCs) are believed to cause strengthening effects, according to previous studies. Here, the representative volume element (RVE) based computational homogenization technique was used to study the tensile deformation of PRMMCs with different particle aspect ratios (AR). The influence of TRSS was assessed quantitatively via comparing simulations with or without the cooling process. It was found that the strengthening effect of TRSS was affected by the particle AR. With the average strengthening effect of TRSS, a fast method of introducing the strengthening effect of TRSS to the tensile behavior of PRMMCs was developed. The new method has reduced the computational cost by a factor 2. The effect of TRSS on continuous fiber-reinforced metal matrix composite was found to have a softening-effect during the entire tensile deformation process because of the pre-yield effect caused by the cooling process.
Thermal conductivity of SiC fine particles reinforced Al alloy matrix composite with dispersed particle size
[J].
Effect of particle size on microstructure and mechanical properties of SiCp/AZ91 magnesium matrix composite
[J].
Simulation of anisotropic load transfer and stress distribution in SiCp/Al composites subjected to tensile loading
[J].
Effect of thermal cycling on the expansion behavior of Al/SiCp composite
[J].
Surface hardening analysis for shot peened GH4720Li superalloy using a DEM-FEM coupling RV simulation method
[J].
A coupling model of element-based peridynamics and finite element method for elastic-plastic deformation and fracture analysis
[J].
Insights into hardening, plastically deformed zone and geometrically necessary dislocations of two ion-irradiated FeCrAl(Zr)-ODS ferritic steels: A combined experimental and simulation study
[J].
Dislocation evolution at a crack-tip in a hexagonal close packed metal under plane-stress conditions
[J].
Suppressed negative effects of natural aging by pre-aging in SiCp/6092Al composites
[J].
Residual stress and distortion during quench hardening of steels: A review
[J].
Cryogenic treatment of metal—A review
[J].